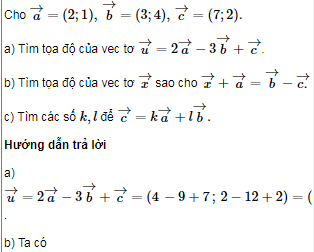
Giải bài 23 trang 24 Sách giáo khoa (SGK) Hình học 10 Nâng cao

Chia sẻ trang này

Gọi M và N lần lượt là trung điểm các đoạn thẳng AB và CD. Chứng minh rằng

Bài 30. Gọi \(M\) và \(N\) lần lượt là trung điểm các đoạn thẳng \(AB\) và \(CD\). Chứng minh rằng

\(2\overrightarrow {MN} = \overrightarrow {AC} + \overrightarrow {BD} = \overrightarrow {AD} + \overrightarrow {BC} .\)

Hướng dẫn trả lời

Theo quy tắc ba điểm, ta có

\(\eqalign{
& \overrightarrow {AC} + \overrightarrow {BD} = \left( {\overrightarrow {AM} + \overrightarrow {MN} + \overrightarrow {NC} } \right) + \left( {\overrightarrow {BM} + \overrightarrow {MN} + \overrightarrow {ND} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \left( {\overrightarrow {AM} + \overrightarrow {BM} } \right) + \left( {\overrightarrow {NC} + \overrightarrow {ND} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \overrightarrow 0 + \overrightarrow 0 = 2\overrightarrow {MN} \cr
& \overrightarrow {AD} + \overrightarrow {BC} = \left( {\overrightarrow {AM} + \overrightarrow {MN} + \overrightarrow {ND} } \right) + \left( {\overrightarrow {BM} + \overrightarrow {MN} + \overrightarrow {NC} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \left( {\overrightarrow {AM} + \overrightarrow {BM} } \right) + \left( {\overrightarrow {NC} + \overrightarrow {ND} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \overrightarrow 0 + \overrightarrow 0 = 2\overrightarrow {MN} \cr} \)

Vậy \(2\overrightarrow {MN} = \overrightarrow {AC} + \overrightarrow {BD} = \overrightarrow {AD} + \overrightarrow {BC} .\)

Trên đây là bài học "Giải bài 23 trang 24 Sách giáo khoa (SGK) Hình học 10 Nâng cao" mà dayhoctot.com muốn gửi tới các em. Để rèn luyện về kỹ năng làm bài thi và kiểm tra các em tham khảo tại chuyên mục "Đề thi học kì 1 lớp 10" nhé.

Nếu thấy hay, hãy chia sẻ tới bạn bè để cùng học và tham khảo nhé! Và đừng quên xem đầy đủ các bài Giải bài tập Toán Lớp 10 của dayhoctot.com.