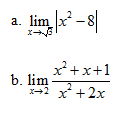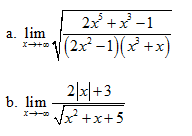
Giải câu 23 trang 152 SGK Đại số và Giải tích 11 Nâng cao

Bài trước
Bài sau  

Tìm các giới hạn sau:

Bài 23. Tìm các giới hạn sau :

a.  \(\mathop {\lim }\limits_{x \to 2} \left( {3{x^2} + 7x + 11} \right)\)

b.  \(\mathop {\lim }\limits_{x \to 1} {{x - {x^3}} \over {\left( {2x - 1} \right)\left( {{x^4} - 3} \right)}}\)

c.  \(\mathop {\lim }\limits_{x \to 0} x\left( {1 - {1 \over x}} \right)\)

d.  \(\mathop {\lim }\limits_{x \to 9} {{\sqrt x - 3} \over {9x - {x^2}}}\)

e.  \(\mathop {\lim }\limits_{x \to \sqrt 3 } \left| {{x^2} - 4} \right|\)

f.  \(\mathop {\lim }\limits_{x \to 2} \sqrt {{{{x^4} + 3x - 1} \over {2{x^2} - 1}}} \)

Giải:

a.  \(\eqalign{& \mathop {\lim }\limits_{x \to 2} \left( {3{x^2} + 7x + 11} \right) = \mathop {\lim }\limits_{x \to 2} 3{x^2} + \mathop {\lim }\limits_{x \to 2} 7x + \mathop {\lim }\limits_{x \to 2} 11 \cr & = {3.2^2} + 7.2 + 11 = 37 \cr} \)

b.  \(\mathop {\lim }\limits_{x \to 1} {{x - {x^3}} \over {\left( {2x - 1} \right)\left( {{x^4} - 3} \right)}} = {0 \over { - 2}} = 0\)

c.  \(\mathop {\lim }\limits_{x \to 0} x\left( {1 - {1 \over x}} \right) = \mathop {\lim }\limits_{x \to 0} \left( {x - 1} \right) = - 1\)

d.  \(\mathop {\lim }\limits_{x \to 9} {{\sqrt x - 3} \over {9x - {x^2}}} = \mathop {\lim }\limits_{x \to 9} {{\sqrt x - 3} \over { - x\left( {x - 9} \right)}} = - \mathop {\lim }\limits_{x \to 9} {1 \over {x\left( {\sqrt x + 3} \right)}} = - {1 \over {54}}\)

e.  \(\mathop {\lim }\limits_{x \to \sqrt 3 } \left| {{x^2} - 4} \right| = 1\)

f.  \(\mathop {\lim }\limits_{x \to 2} \sqrt {{{{x^4} + 3x - 1} \over {2{x^2} - 1}}} = \sqrt {{{{2^4} + 3.2 - 1} \over {{{22}^2} - 1}}} = \sqrt 3 \)

Bài trước
In bài này
Bài sau  
Các bài học liên quan
Các chương học và chủ đề lớn
Học tốt các môn khác lớp 11

Bài học nổi bật nhất

Đề thi lớp 11 mới cập nhật