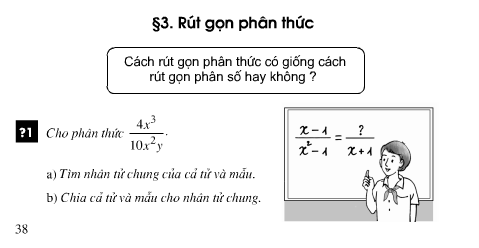
Giải bài 7 trang 39 sách giáo khoa toán 8 tập 1
Chia sẻ trang này
Bài 7. Rút gọn phân thức:
- Bài học cùng chủ đề:
- Bài 8 trang 40 sách giáo khoa toán 8 tập 1
- Bài 9 trang 40 sách giáo khoa toán 8 tập 1
- Bài 10 trang 40 sách giáo khoa lớp 8 tập 1
- Ngữ pháp tiếng anh hay nhất
Bài 7. Rút gọn phân thức:
a) \( \frac{6x^{2}y^{2}}{8xy^{5}}\); b) \( \frac{10xy^{2}(x + y)}{15xy(x + y)^{3}}\);
c) \( \frac{2x^{2} + 2x}{x + 1}\); d) \( \frac{x^{2}- xy - x + y}{x^{2} + xy - x - y}\)
Giải
a) \( \frac{6x^{2}y^{2}}{8xy^{5}}= \frac{3x.2xy^{2}}{4y^{3}.2xy^{2}}= \frac{3x}{4y^{3}}\)
b) \( \frac{10xy^{2}(x + y)}{15xy(x + y)^{3}} = \frac{2y.5xy(x + y)}{3(x + y)^{2}.5xy(x + y)}= \frac{2y}{3(x + y)^{2}}\)
c) \( \frac{2x^{2} + 2x}{x + 1}= \frac{2x(x + 1)}{x + 1} = 2x\)
d) \( \frac{x^{2}- xy - x + y}{x^{2} + xy - x - y}= \frac{x(x - y)- (x - y)}{x(x + y)- (x + y)}= \frac{(x - y)(x - 1)}{(x + y)(x - 1)} = \frac{x - y}{x + y}\)
Chia sẻ trang này
Các bài học liên quan
Các chương học và chủ đề lớn
