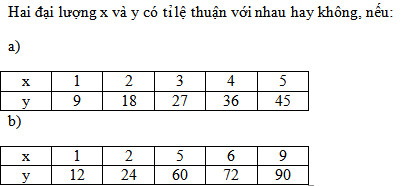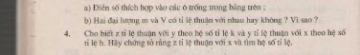
Giải bài 102 trang 50 sgk toán 7 tập 1
Chia sẻ trang này
Từ tỉ lệ thức
Bài 102. Từ tỉ lệ thức : \({a \over b} = {c \over d}\left( {a,b,c,d \ne 0;a \ne \pm b;c \ne \pm d} \right)\), hãy suy ra các tỉ lệ thức sau:
a) \({{a + b} \over b} = {{c + d} \over d}\) b) \({{a - b} \over b} = {{c - d} \over d}\)
c) \({{a + b} \over a} = {{c + d} \over c}\) d) \({{a - b} \over a} = {{c - d} \over c}\)
e) \({a \over {a + b}} = {c \over {c + d}}\) f) \({a \over {a - b}} = {c \over {c - d}}\)
Giải
a) \({a \over b} = {c \over d} \Rightarrow {a \over c} = {b \over d} \Rightarrow {a \over c} = {b \over d} = {{a + b} \over {c + d}}\)
Từ: \({{a + b} \over {c + d}} = {b \over d} \Rightarrow {{a + b} \over b} = {{c + d} \over d}\)
b) \({a \over b} = {c \over d}\Rightarrow {a \over c} = {b \over d}\Rightarrow {a \over c} = {b \over d} = {{a - b} \over {c - d}}\)
Từ: \({{a - b} \over {c - d}} = {b \over d} \Rightarrow {{a - b} \over b} = {{c - d} \over d}\)
c) \({a \over b} = {c \over d}\Rightarrow {a \over c} = {b \over d} = {{a + b} \over {c + d}}\)
Từ: \({{a + b} \over {c + d}} = {a \over c}\Rightarrow {{a + b} \over a} = {{c + d} \over c}\)
d) \({a \over b} = {c \over d} \Rightarrow {a \over c} = {b \over d} = {{a - b} \over {c - d}}\)
Từ: \({{a - b} \over {c - d}} = {a \over c} \Rightarrow {{a - b} \over a} = {{c - d} \over c}\)
e) \({a \over b} = {c \over d} \Rightarrow {a \over c} = {b \over d} = {{a + b} \over {c + d}}\)
Từ: \({a \over c} = {{a + b} \over {c + d}} \Rightarrow {a \over {a + b}} = {c \over {c + d}}\)
f) \({a \over b} = {c \over d}\Rightarrow {a \over c} = {b \over d} = {{a - b} \over {c - d}}\)
\({a \over c} = {{a - b} \over {c - d}} \Rightarrow {a \over {a - b}} = {c \over {c - d}}\)
Chia sẻ trang này
Các bài học liên quan
Các chương học và chủ đề lớn
